X y=2 2x 2y=4 solution 205163

Which of the following pair of linear equations has unique solution, no solution or infinitely many solutions In case there is a unique solution, find it by using cross multiplication method x y = 2 2x 2yGraphical Method of Solution of a Pair of Linear Equations video tutorialNote that we had to add 2y on both sides of the equation to get that result Substitute the value of x into the other equation so that you get an expression in terms of y So y2 − x2 = 2x 4 becomes y2 −(2 2y)2 = 2(2 2y) 4 ⇒ y2 − (4 8y 4y2) = 4 4y 4

1

X y=2 2x 2y=4 solution

X y=2 2x 2y=4 solution-Ex 42, 3 Equation x − 2y = 4 and which are not (i) (0,2) (0,2) Putting x = 0 and y = 2 in LHS LHS = 0 − 2 (2) = − 4 ≠ 4 ∴ Hence, LHS ≠ RHS ∴ Not a solution Ex 42, 3 Equation x − 2y = 4 and which are not (ii) (2,0) (2,0) Putting x = 2 and y = 0 in LHSClick here 👆 to get an answer to your question ️ Determine the number of solutions for systems xy=2 and 2x2y=8 1 Log in Join now 1 Log in Join now Ask your question Awilli25 3 weeks ago Mathematics College 5 pts Answered

Draw The Graph Of The Pair Of Equations 2x Y 4 And 2x Y 4 Studyrankersonline

In mathematics, particularly in algebra, an indeterminate system is a system of simultaneous equations (eg, linear equations) which has more than one solution (sometimes infinitely many solutions) In the case of a linear system, the system may be said to be underspecified, in which case the presence of more than one solution would imply an infinite number of solutions (sinceHow many solutions does the system of equations have?Solution for 6 y= 2x1 2x2y = 4 A No solution B Infinitely Many Solutions C (1,1) D (0, 6) 7 y=3x 2 y = 2x8 A No Solution B Infinitely Many

Transcribed image text Find the general solution 1 fx' = x 3y ly' = 3x y 2 (x' = 4x y by' = 2x y 3 ('= 2x y y' = 2y 4 Match each of the systems in problems 13 with the appropriate phase pane Draw the eigenspaces for the realvalued eigenvalues State whether the equilibrium point is stable or not ExplainHi, Showing graphically x^2y^22x2y=0 completing squares x^22x y^22y =0 (x1)^2 1 (y1)^2 1 = 0 (x1)^2 (y1)^2 = 2 x^2y^24x6y12=0 completing squaresSee a solution process below Step 1) Solve the first equation for x 1/2x 2y = 4 color(red)(2)(1/2x 2y) = color(red)(2) * 4 (color(red)(2) * 1/2x) (color(red

M 2y=8 M 2x y = 4 x y = 2 8 L * y 4 9 L 2x 3y = 8 10 L M 2x 2y = 4 4x 2y = 7 M 3x 3y = 12 M 6x 9y = 2 M 2x y = 2 11Simple and best practice solution for 2xy=4;x2y=2 Check how easy it is, to solve this system of equations and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework If it's not what You are looking for type in the system of equations solver your own equations and let us solve itY=1/2x3 x2y=6 a one b two c infinitely many d none 3 How many math How many solutions does the system have?

Find The Asymptotes Of The Following Curves Y 3 X 2y 2x Y 2 2x

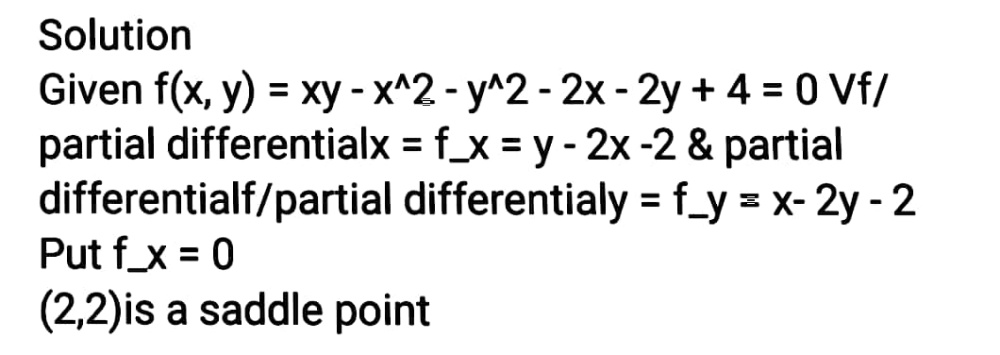
Which Of The Followings Is Correct For The Functio Gauthmath

Notice 2y=42x Is just bb(y=x2) multiplied by bb2 2(y=x2) In this situation we have to assign an arbitrary value to one of the variables and express the other variable in terms of this So for arbitrary x y=x2 We can write the solutions as (x,y)>(x,x2) Since we are assigning a value to x and calculating the corresponding value of yTry solving for one of the variables first, and then the other;X2y=4 b) 2xy=1 multiply the equation by 2

Solution Graph 2x 2y 4 By Plotting Points The Rule Of Thumb Suggests Plotting 3 Points And Then Draw A Line So Plot At Least 3 Points Draw An Accurate And Straight Line

Draw The Graph Of The Pair Of Equations 2x Y 4 And 2x Y 4 Write The Vertices Of The Triangle Youtube

Y x = 2 2y 2x = 4 Solve by Substitution // Solve equation 1 for the variable y 1 y = x 2 // Plug this in for variable y in equation 2 2 2x 2• (x 2) = 4 2 4x = 8 // Solve equation 2 for the variable x 2 4x = 8 2 x = 2 // By now we know this much x = 2 y = x 2Precalculus Matrix Row Operations Solving a System of Equations Using a Matrix 1 Answer Alan P How do I use matrices to find the solution of the system of equations #y=−2x−4# and #y4=−2x#?The quadratic formula gives two solutions, one when ±

Activity3 Graphing Syste Descubre Como Resolverlo En Qanda

Draw The Graphs Of The Pair Of Linear Equations X Y 2 0 Amp 4x Y 4 0 Determine The Co Ordinates Of The Vertices Of The Triangle Formed By The Lines

Click here 👆 to get an answer to your question ️ x=2, y=1 is a solution of the linear equation A x2y=0 B x2y=4 C 2xy=0 D 2xy=5Solve by Substitution 2xy=10 2x2y=4 2x − y = 10 2 x y = 10 2x − 2y = 4 2 x 2 y = 4 Solve for y y in the first equation Tap for more steps Subtract 2 x 2 x from both sides of the equation − y = 10 − 2 x y = 10 2 x 2 x − 2 y = 4 2 x 2 y = 4 Multiply each term in − y = 10 − 2 x y = 10 2 xDivide both sides by 2 Divide both sides by − 2 \frac {2y} {2}=\frac {2x4} {2} − 2 − 2 y = − 2 − 2 x − 4 Dividing by 2 undoes the multiplication by 2 Dividing by − 2 undoes the multiplication by − 2 y=\frac {2x4} {2} y = − 2 − 2 x − 4 Divide 2x4 by 2

Answered Find The Solution Of The Following Bartleby

Differential Equations Solved Examples Show That Following Differential Equation Is Not Exact 3x 2y 4 2xy Dx 2x 3y 3 X 2 Dy 0 Then Find An Integrating Factor To Solve The Differential Equation

Equations are 2x 2y = 4 3y x = 1 presumably you want to solve them simultaneously use the second equation to solve for y in terms of x and then use that value to substitute in the first equation to solve for x second equation is 3y x = 1 add x to both sides and subtract 1 from both sides to getA Power consists of two parts, ((2x^2)/(3y^3))^3(y^2/x^3)^2(x/(2y))^4 Exponent and Base, We are going to learn to identify components of a Power and to evaluate itThis is an example of simultaneous equations These may be solved by eliminating a variable as follows a) x2y=4 multiply the equation by 1 ==>

Http Mansfieldalgebra1 Weebly Com Uploads 1 0 4 4 Rc 2 Answer Key Pdf

Solution Finding X Y The Questionis X 2y 4 2x Y 3 I Don 39 T Understand When Finding 2x

Algebra Graph 2x2y=4 2x − 2y = 4 2 x 2 y = 4 Solve for y y Tap for more steps Subtract 2 x 2 x from both sides of the equation − 2 y = 4 − 2 x 2 y = 4 2 x Divide each term by − 2 2 and simplify Tap for more stepsXy= 2 1 2x2y=4 /2 xy=2 2 xy= 2 xy= 2 Add the two equations 2x=4 /2 x=2 plug value of x in (1)1 y x = 2 2 2y 2x = 4 Graphic Representation of the Equations x y = 2 2x 2y = 4 Solve by Substitution // Solve equation 1 for the variable y 1 y = x 2 // Plug this in for variable y in equation 2 2 2• (x 2) 2x = 4 2 4x = 0 // Solve

Math 432 Hw 2 5 Solutions

1

Let f(x,y) = xy x^2 y^2 2x 2y 4 Find the gradient delta f(1,2) delta f(x,y) = (fx, fy) Fx = y 2x 2 Fy = x 2y 2 delta f(1,2) = ( y 2x 2, x 2y 2) delta f(1,2) = ( 2, 5) Find the directions of that Du f(1,2) = 0 u = (5,2) D = delta f(1,2) uYou can put this solution on YOUR website!Algebra Graph x2y=4 x − 2y = 4 x 2 y = 4 Solve for y y Tap for more steps Subtract x x from both sides of the equation − 2 y = 4 − x 2 y = 4 x Divide each term by − 2 2 and simplify Tap for more steps

Which Of The Following Pairs Of Linear Equations Are Consistent Inconsistent If Consistent Obtain The Solution Graphically I X Y 5 2x 2y 10 Ii X Y 8

Solve The Following Systems Of Equations Graphically X Y 6 X Y 2 Sarthaks Econnect Largest Online Education Community

Simple and best practice solution for x^22x1y^22y1=4 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework If it's not what You are looking for type in the equation solver your own equation and let us solve itTranscribed image text Apply the method of undetermined coefficients to find a particular solution to the following system x' = 2x y 2 el y' = x 2y 4 et Xp(t) = 0 Apply the method of undetermined coefficients to find a particular solution to the following system x' = 3x 2y 3 e' y' = 2x 3y 2 et Non Xp (t) = Itelt 4 el NOGet stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!

Let S Draw Graphs Of X Y 4 2x Y 2 And Observe Them Sarthaks Econnect Largest Online Education Community

Solve 3x Y 2x Y 2 9x Y 4x Y 1

Graphical Method of Solution of a Pair of Linear Equations video tutorial ;2x2y=4 Geometric figure Straight Line Slope = 1 xintercept = 2/1 = 0000 yintercept = 2/1 = 0000 Rearrange Rearrange the equation by subtracting what is to the right of theIt also can be solved by elimination method but we will be mostly focusing on substitution method Now, let's suppose the equation xy=2 as eq'n 1 and 2xy=4 as eq'n 2 Solution in equation 2 2xy=4 2x=y4 x= (y4)/2 Now substituting value of x

Engineering Mathematics Notes

Differential Equations Solved Examples Show That Following Differential Equation Is Not Exact 3x 2y 4 2xy Dx 2x 3y 3 X 2 Dy 0 Then Find An Integrating Factor To Solve The Differential Equation

Solution for x^22xy^22y=4 equation Simplifying x 2 2x y 2 2y = 4 Reorder the terms 2x x 2 2y y 2 = 4 Solving 2x x 2 2y y 2 = 4 Solving for variable 'x' Reorder the terms 4 2x x 2 2y y 2 = 4 4 Combine like terms 4 4 = 0 4 2x x 2 2y y 2 = 0 The solution to this equation could not be determinedQuestion If X2 Y2 = 28 And 2x 2y = 4, Then What Is X?You can put this solution on YOUR website!

Answered Which Of The Following Is The General Bartleby

How To Evaluate Lim X Y 1 1 Xy Y 2x 2 X Y Quora

Steps for Solving Linear Equation ( 2 x y ^ { 2 } 3 ) d x ( 2 x ^ { 2 } y 4 ) d y = 0 ( 2 x y 2 − 3) d x ( 2 x 2 y 4) d y = 0 Use the distributive property to multiply 2xy^ {2}3 by d Use the distributive property to multiply 2 x y 2 − 3 by d \left (2xy^ {2}d3d\right)x\left (2x^ {2}y4\right)dy=0Let's start by putting the two equations in the same form y = mx b This can be done by dividing both sides of the first equation by 2 2y = −4x 4 → y = −4x 4 2 → y = 2(− 2x 2) 2 → y = − 2x 2 The two lines have the same equation, hence there are infinite solutions (since the two lines lie one on top of one another)X2y=4 2x4y=12 Step 1 Let's put the above equations in slopeintercept form given as y=mxb where m is the slope and b is the yintercept when x=0 or at point (0,b) Step 2 Let's start with Add 2y4 to both sides of the equation Divide 2 to both sides of the equation or Step 3

What Is The Solution Of The Differential Equation Math Xy 3 Y Dx 2 X 2y 2 X Y 4 Dy 0 Math Quora

Differential Equatio Long Answer Questions 7 M R Solye 2x Y 3 Dx 2y X 1 Dy Sol Dy 2x Y 3 Given Equation Is

Graphical Method of Solution of a Pair of Linear Equations video tutorial ;Solution for 2 (2xy)=2 (xy)4 equation Simplifying 2 (2x 1y) = 2 (x y) 4 (2x * 2 1y * 2) = 2 (x y) 4 (4x 2y) = 2 (x y) 4 4x 2y = (x * 2 y * 2) 4 4x 2y = (2x 2y) 4 Reorder the terms 4x 2y = 4 2x 2y Solving 4x 2y = 4 2x 2y Solving for variable 'x'Answer by funmath (2933) ( Show Source ) You can put this solution on YOUR website!

What Is The Solution Of Math X Y 2 2y 2 27 Math Where X And Y Are Integers Quora

1

Graphical Method of Solution of a Pair of Linear Equations video tutorial ;You can put this solution on YOUR website!Graph x^2y^22x2y1=0 Find the standard form of the hyperbola Tap for more steps Add to both sides of the equation Complete the square for Tap for more steps Use the form , to find the values of , , and Consider the vertex form of a parabola Substitute the

Solve The Pair Of Linear Equationd X Y 2 And X Y 4 Brainly In

Draw The Graph Of The Pair Of Equations 2x Y 4 And 2x Y 4 Studyrankersonline

Step by step solution of a set of 2, 3 or 4 Linear Equations using the Substitution Method x2y=4;2xy=2 Tiger Algebra Solver1 y=2x1 2 2x2y=4 Equations Simplified or Rearranged 1 y 2x = 1 2 2y 2x = 4 Graphic Representation of the Equations 2x y = 1 2x 2y = 4 Solve by Substitution // Solve equation 1 for the variable y 1 y = 2x 1 // Plug this in for variable y in equation 2You can put this solution on YOUR website!

The Solution Of The Differential Equation Y Xy 2x 2y 2 Dx X Xy X 2y 2 Dy 0 Is Given Youtube

The Substitution Method

Subtract x from both sides Subtract x from both sides 2y=4x 2 y = 4 − x Divide both sides by 2 Divide both sides by 2 \frac {2y} {2}=\frac {4x} {2} 2 2 y = 2 4 − x Dividing by 2 undoes the multiplication by 2For solving for both the variables, we need two sets of equations In fact, if we have to determine the value of n variables, then we require at least n equations in those variables If the number of equations is less than n, then we cannot find tIf There Are Two Possible Answers, Then Enter The Larger Of The Two If There Are Two Possible Answers, Then Enter The Larger Of The Two This problem has been solved!

Solve 2 2x Y 1 X 2y 5 9 0 And 9 2x Y 6 X 2y 4 0 Youtube

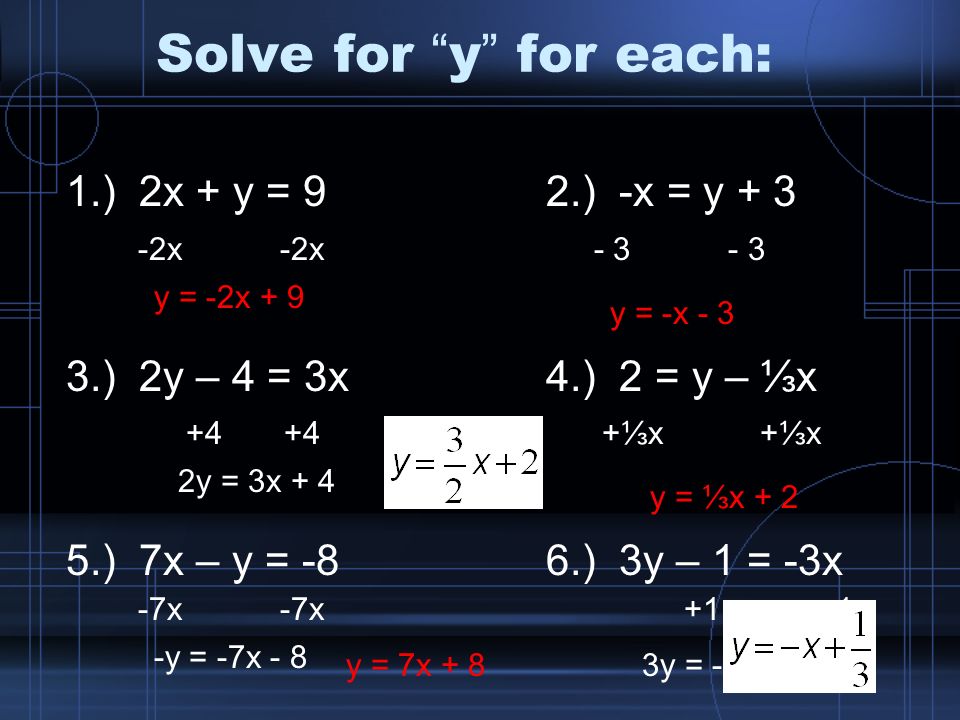
Solve For Y For Each 1 2x Y 9 2 X Y 2y 4 3x 4 2 Y X 5 7x Y 8 6 3y 1 3x 2x 2x Ppt Video Online Download

There are different ways to solve a system of equations In your cases I would say "substitution" is a good way x2y = 4 given x y = 7 given Now in the bold equation above we solve for y y = 7 x so y = x 7 Substitute this into the nonbolL1) x2y=6 L2) y=2x6 Since L2 is already solved for y, plug (2x6) in for y in L1 and solve for x x2 (2x6)=6 x4x12=6 3x12=6 3x1212=612Y 5x = 6 3y 15x = 12 A) infinitely many solutions B) no solution C) two solutions D) one solution

Answers

Graph The System Of Linear Equations Solve The Chegg Com

Is addition and one when it is subtraction All equations of the form a x 2 b x c = 0 can be solved using the quadratic formula 2 a Use calculus to find the area bounded by the circle x^2y^22x2y23=0 and the pair of lines x^22xyy^27x7y12=0How do you solve #x 2y = 4# and #2x y = 3# using matrices?Free math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics homework questions with stepbystep explanations, just like a math tutor

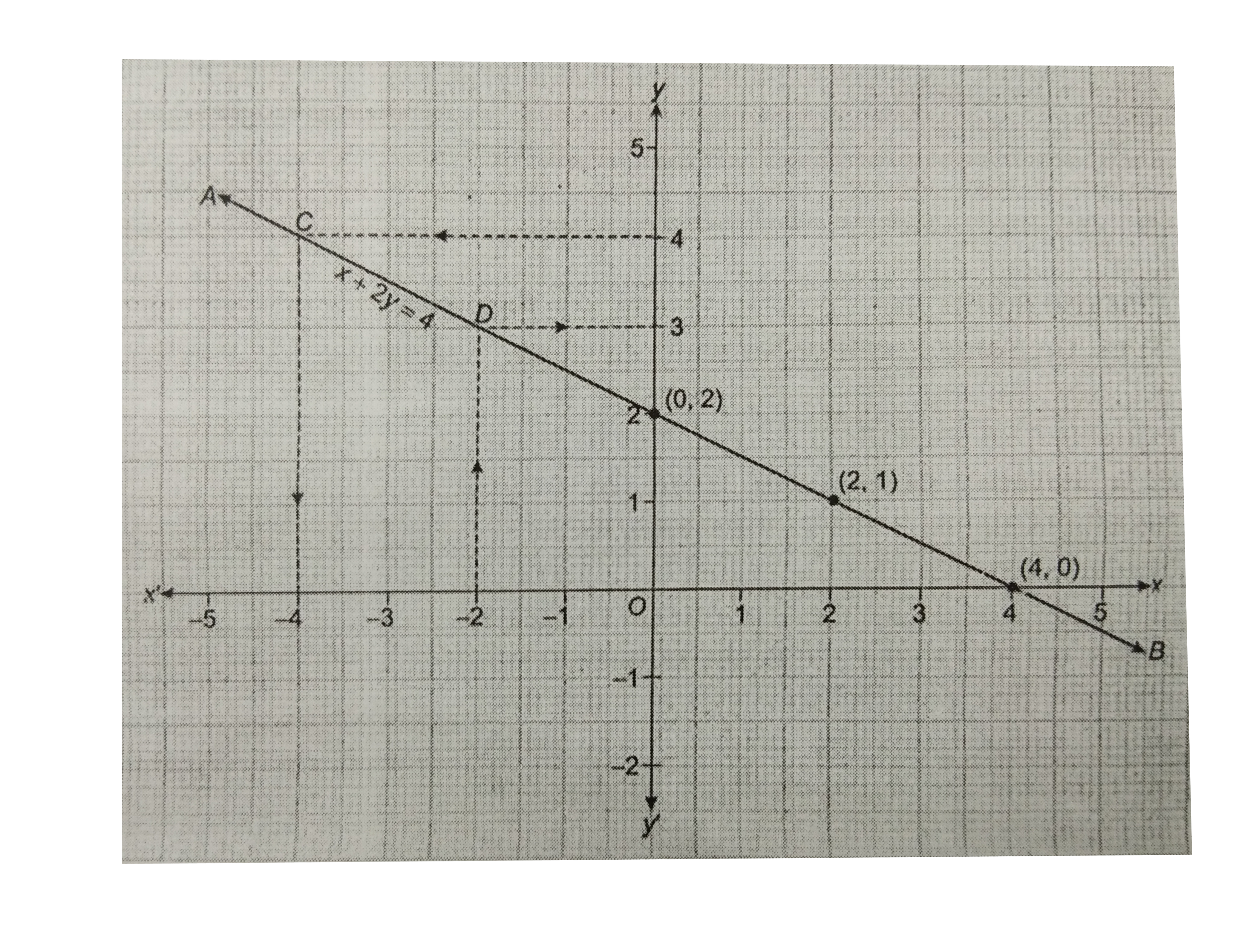
Draw The Graph Of The Equation X 2y 4 Use Graph To Find

Engineering Mathematics Notes

Move all terms containing x to the left, all other terms to the right Add '2y' to each side of the equation 2x 2y 2y = 4 2y Combine like terms 2y 2y = 0 2x 0 = 4 2y 2x = 4 2y Divide each side by '2' x = 2 y Simplifying x = 2 ySolving for the second variable will give you a solid numerical value You can also solve this system of equations using the addition method, which I'll illustrate below 2x 2y = 4

Answered Find The Solution Of The Following Bartleby

Solution How Do You Solve Each System By Graphing 5 2x 2y 4 And Y X 6 6 3x Y 5 And X Y 7 7 5x Y 9 And X 3y 21 8 Y X And Y 5x 0 9 X 10 And X Y 10

Ntercept5 Lntercep5 See How To Solve It At Qanda

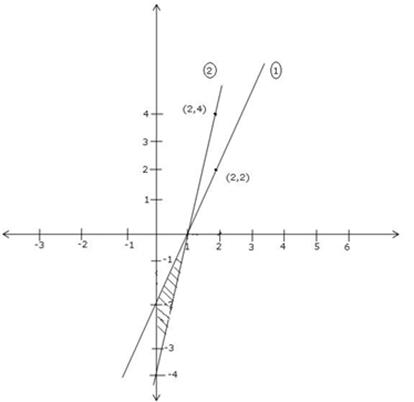
Solve Graphically 2x Y 2 And 4x Y 4 Shade The Region Between These Lines And The Y Axis Mathematics Topperlearning Com 2kj2s63hh

Beginarrayl X Y X Y 2 1 2y 2 2x Y 3 Gauthmath

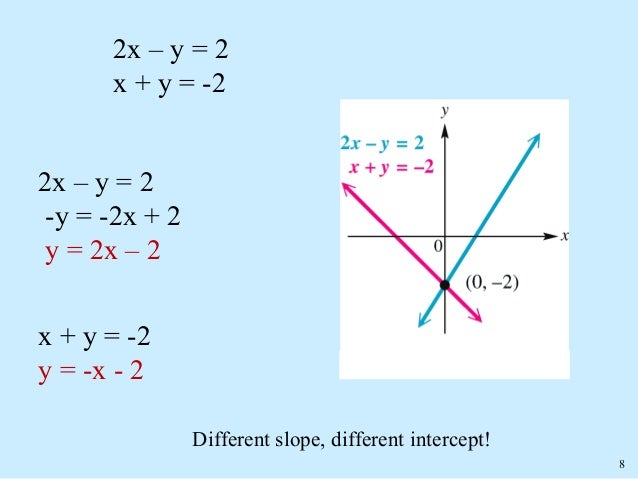
Solving Systems Of Linear Equations

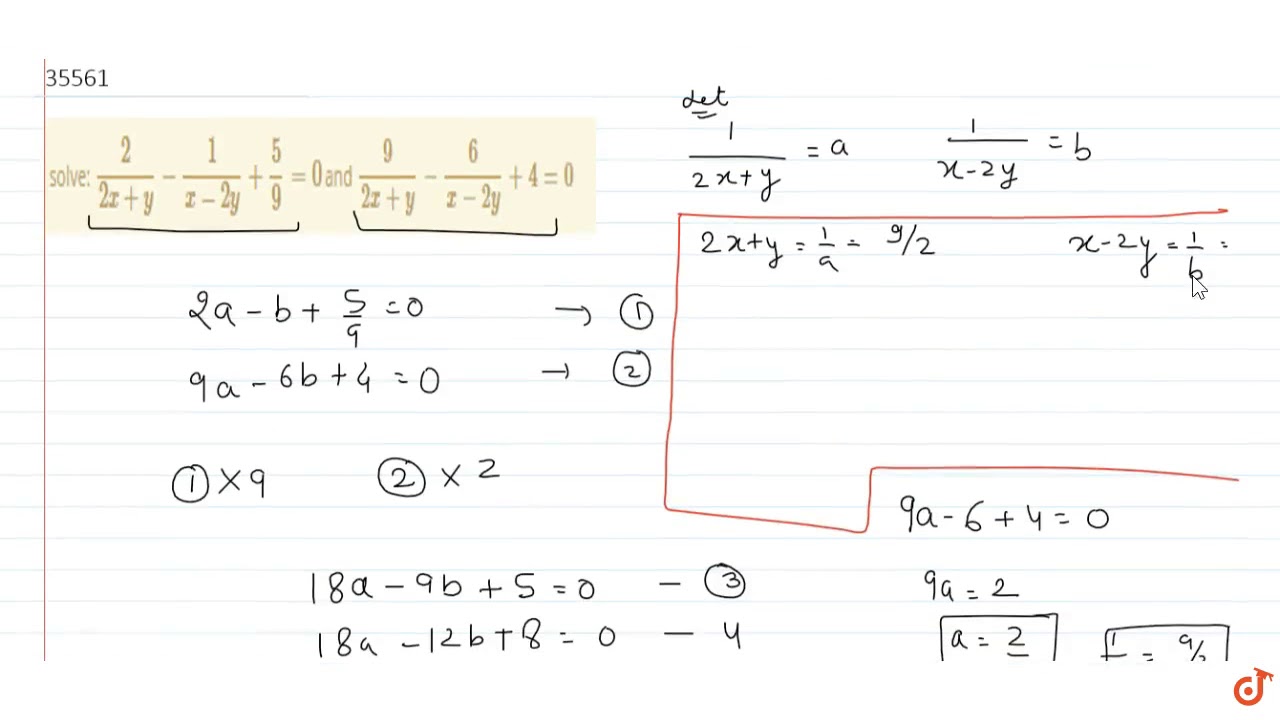
Solve For X And Y 2 2x Y 1 X 2y 5 9 0 9 2x Y 6 X 2y 4 0 Maths Pair Of Linear Equations In Two Variables Meritnation Com

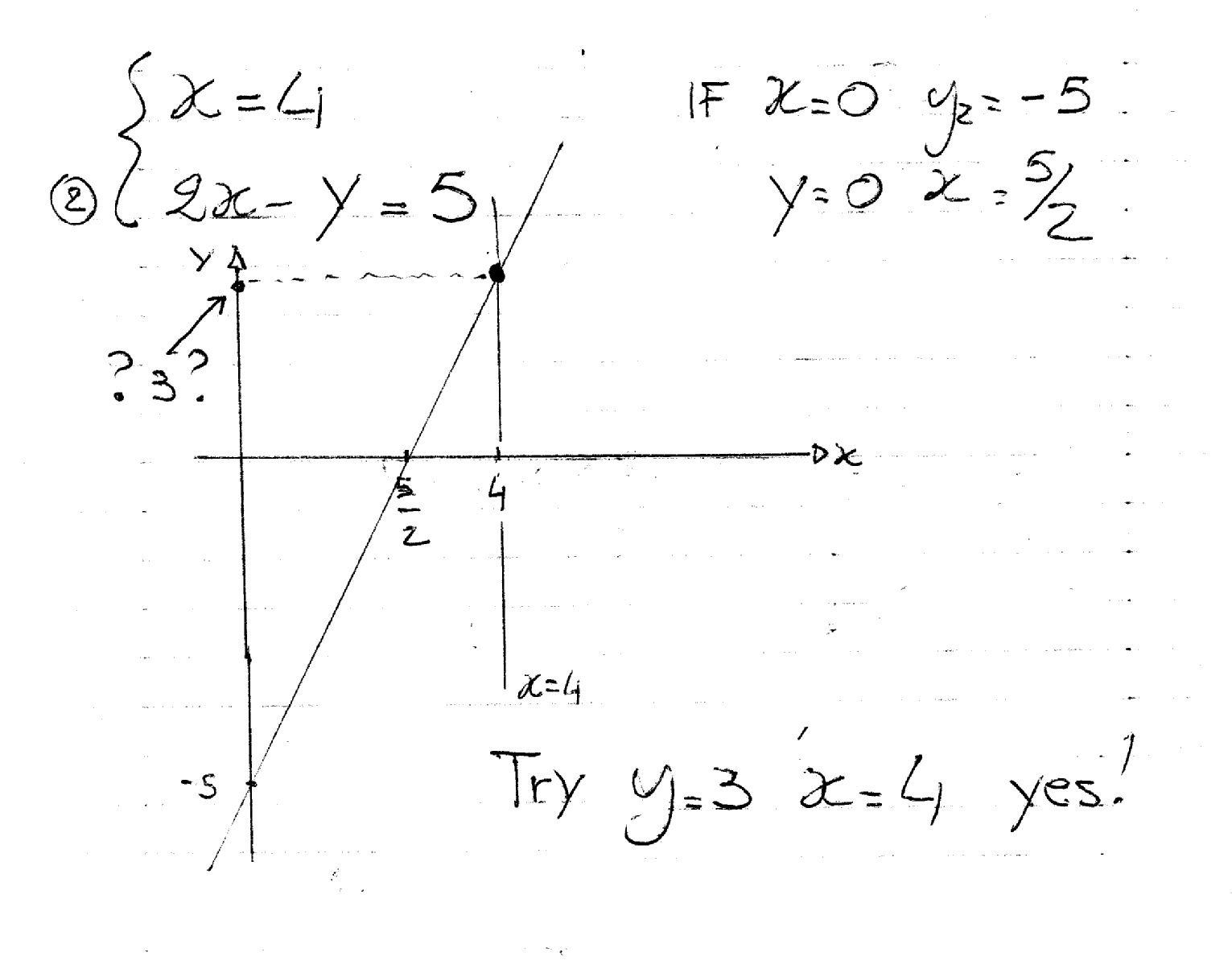
How Do You Solve The System 2x Y 5 And X 4 By Graphing Socratic

Systems Of Equations With Elimination 3y 4x 11 Y 2x 13 Video Khan Academy

Www Tau Ac Il Levant Ode Solution 6 Pdf

If X Y 4 A N D X Y 2 Find The Values Of X 2 Y 2

Example 3 Two Rails Are Represented By X 2y 4 0

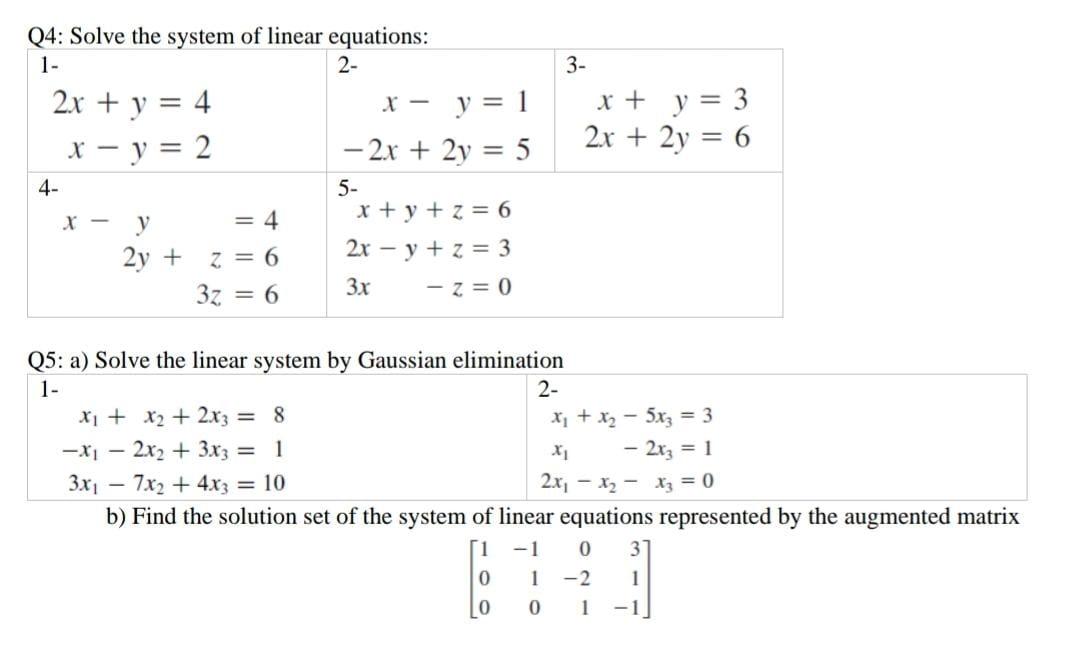
3 X Y 3 2x 2y 6 Q4 Solve The System Of Chegg Com

Exact Equations Example 3 Video Khan Academy

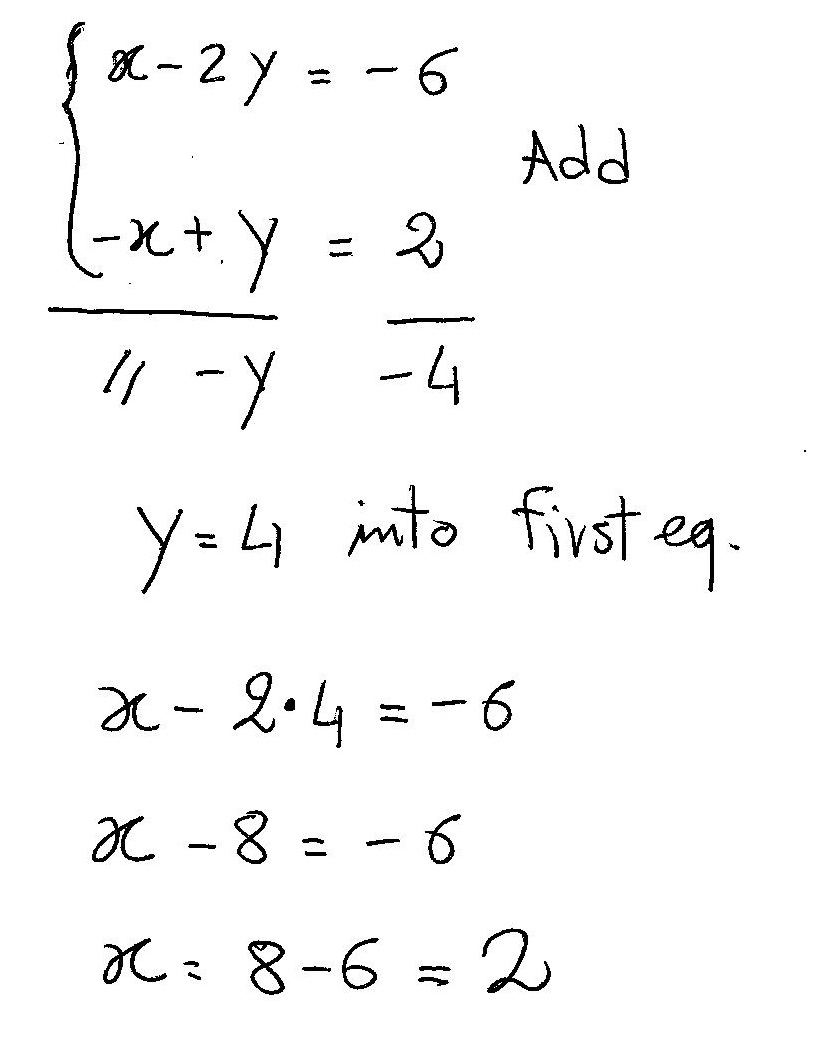
How Do You Solve X 2y 6 And X Y 2 Socratic

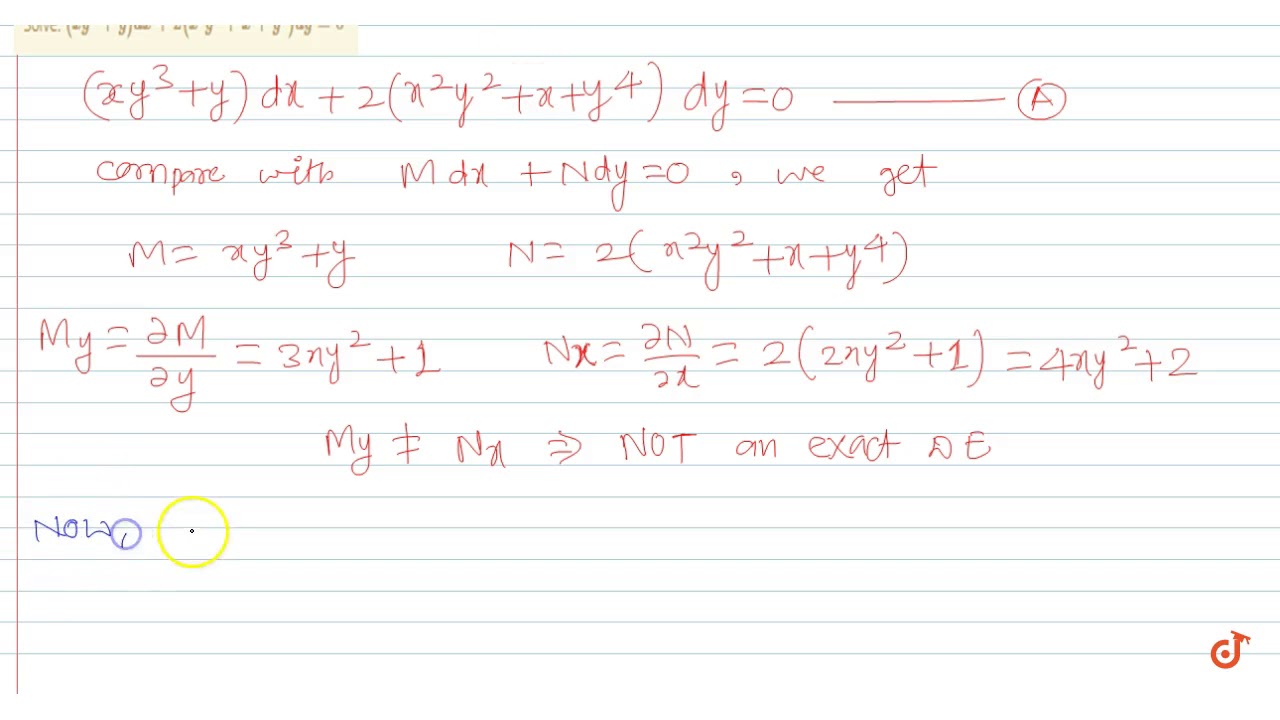
Solve Xy 3 Y Dx 2 X 2y 2 X Y 4 Dy 0 Youtube

What Is The Solution For Math Xy 2 2x 2y 3 Dx X 2y X 3y 2 Dy 0 Math Quora

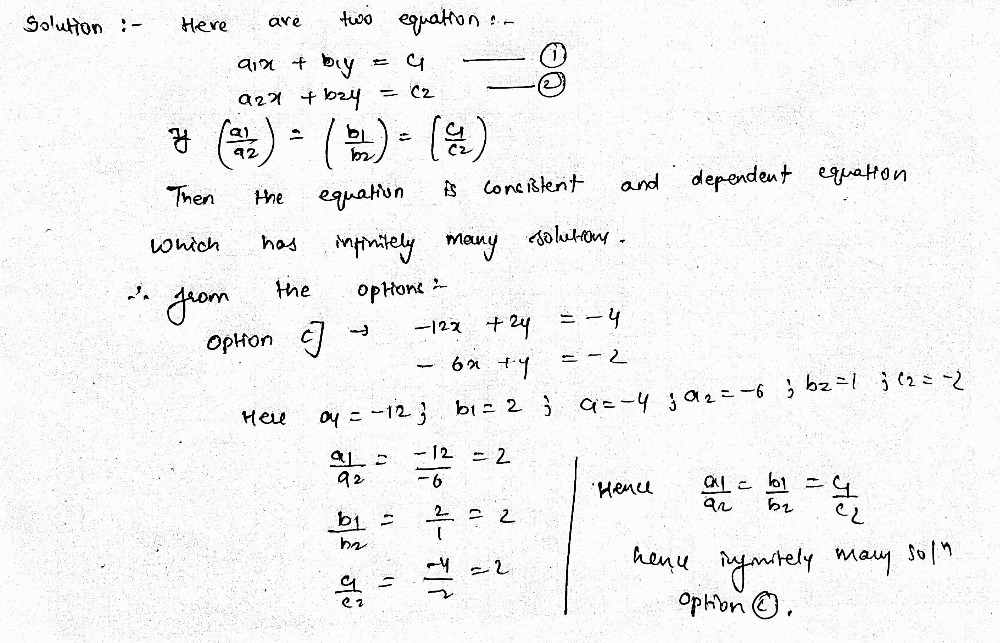
Which System Has Infinitely Many Solutions 3x Y Gauthmath

Q Tbn And9gcrd6b53q9mnb3e3tl2g71xop4nv3bmrbuscz6duiph5wuvpl1ta Usqp Cau

Solve For X And Y 2x Y 6 And X Y 2 Brainly In

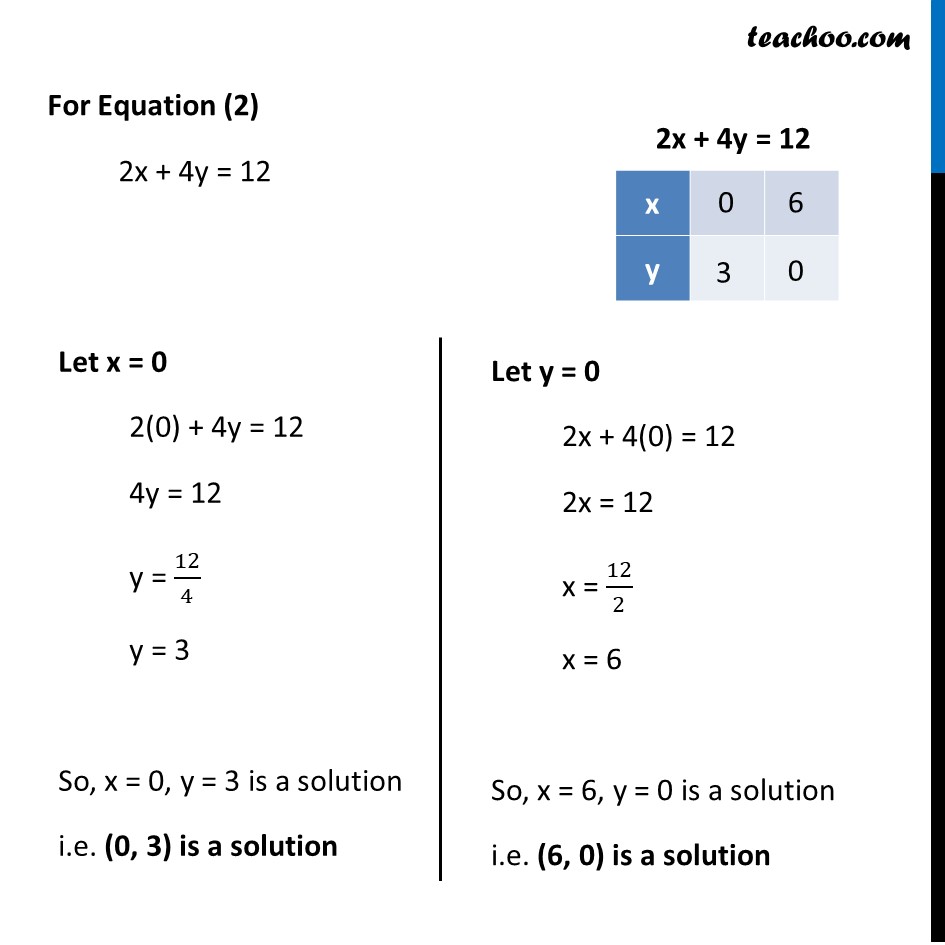
Example 3 Two Rails Are Represented By X 2y 4 0

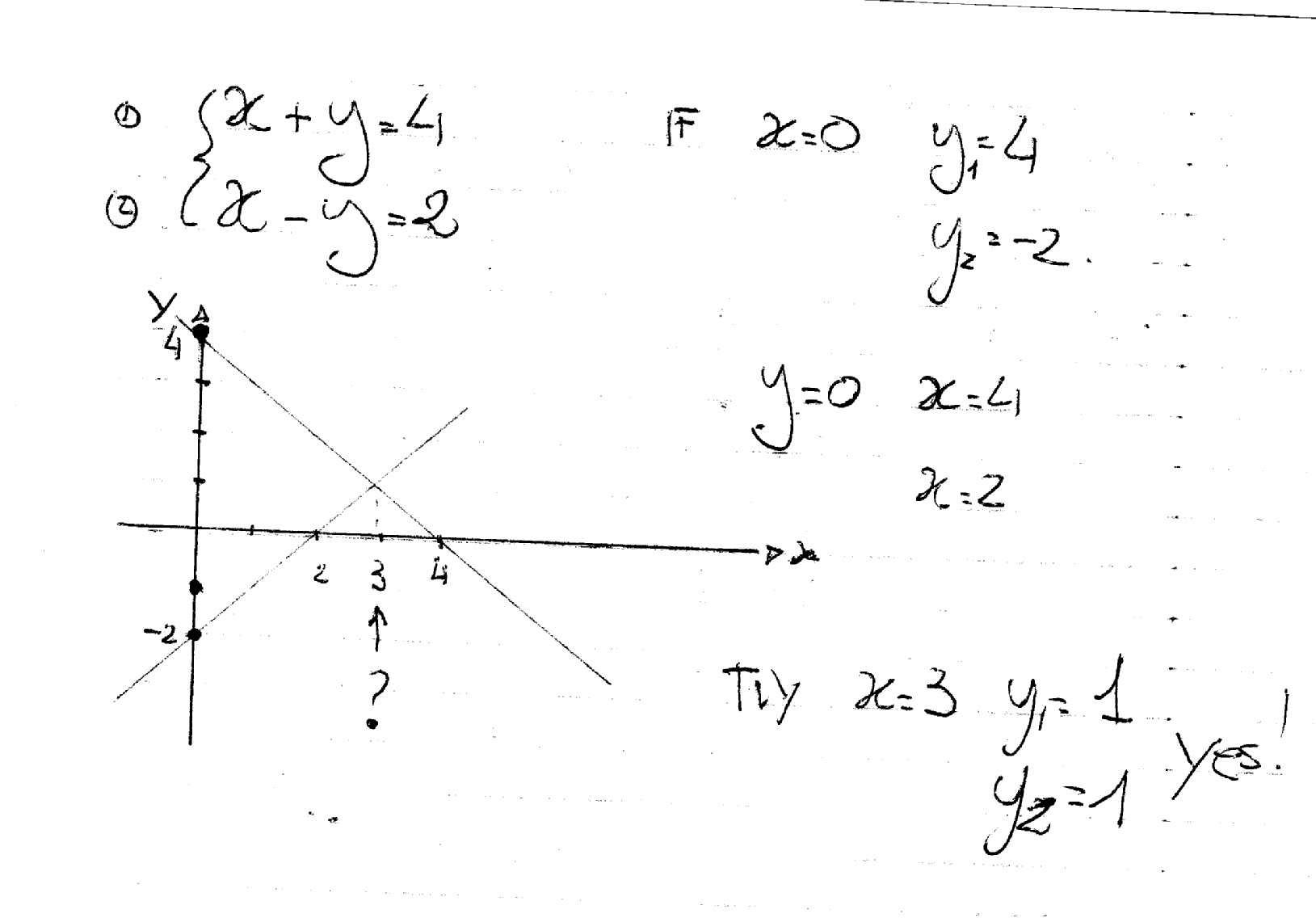
How Do You Solve X Y 4 And X Y 2 By Graphing Socratic

Sketch The Region Enclosed By 2y 4 Sqrt X Y 4 And 2y 2x 6 Decide Whether To Integrate With Respect To X Or Y And Then Find The Area

How Do You Solve The System X 2y 4 And 2x Y 8 By Substitution Socratic

Draw The Graph Of The Pair Of Equations 2x Y 4 And 2x Y 4 Studyrankersonline

Implicit Differentiation

Use The Echelon Method To Solve Each System Of Two Chegg Com

10 X Y 2 X Y 4 And 15 X Y 5 X Y 2 P S 1 4 Q2 Linear Equation In Two Variables Youtube

The Solution Of The Equation X Y 2 And X Y 4 Is Brainly In

Warm Up 4 1 Evaluate 3x 5y For X 3 And Y 4 11 Answer Ppt Download

Find The Products A 3x 2y 4 X X Y 2 Brainly In

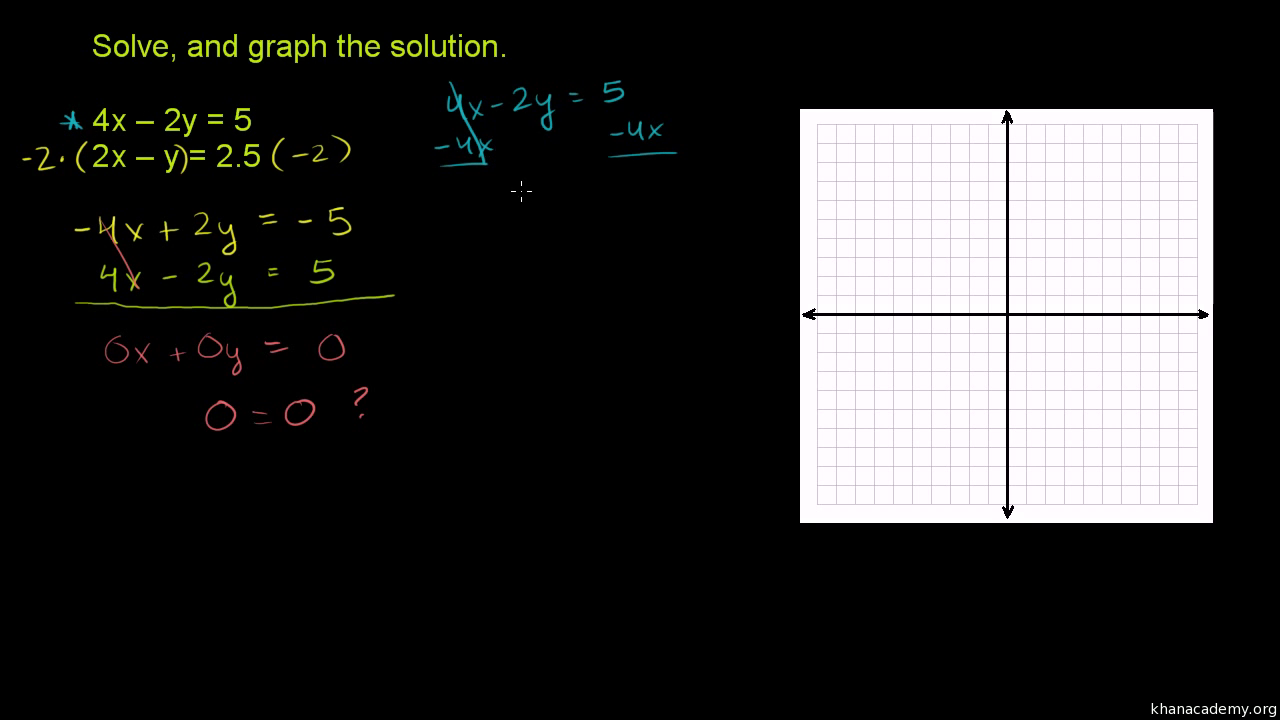
Systems Of Equations With Elimination 4x 2y 5 2x Y 2 5 Video Khan Academy

Graph Graph Inequalities With Step By Step Math Problem Solver

Ii Solve The Following Pairs Ofsirnultaneous Gauthmath

Ex 4 2 3 Which Are Solutions Of X 2y 4 I 0 2 Ii 2 0

Simultaneous Equations Gmat Math Study Guide

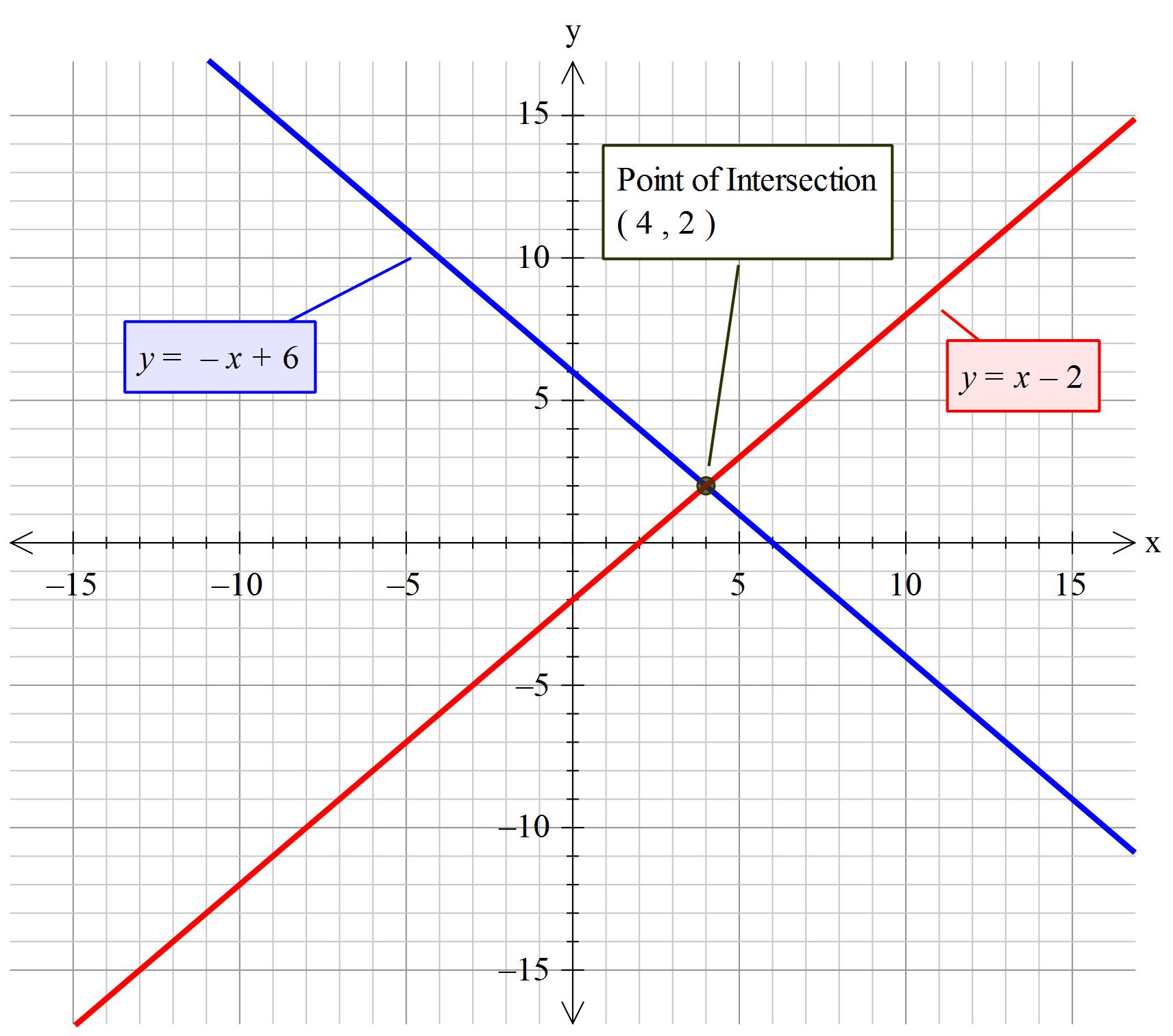
How Do You Solve The System X Y 6 And X Y 2 By Graphing Socratic

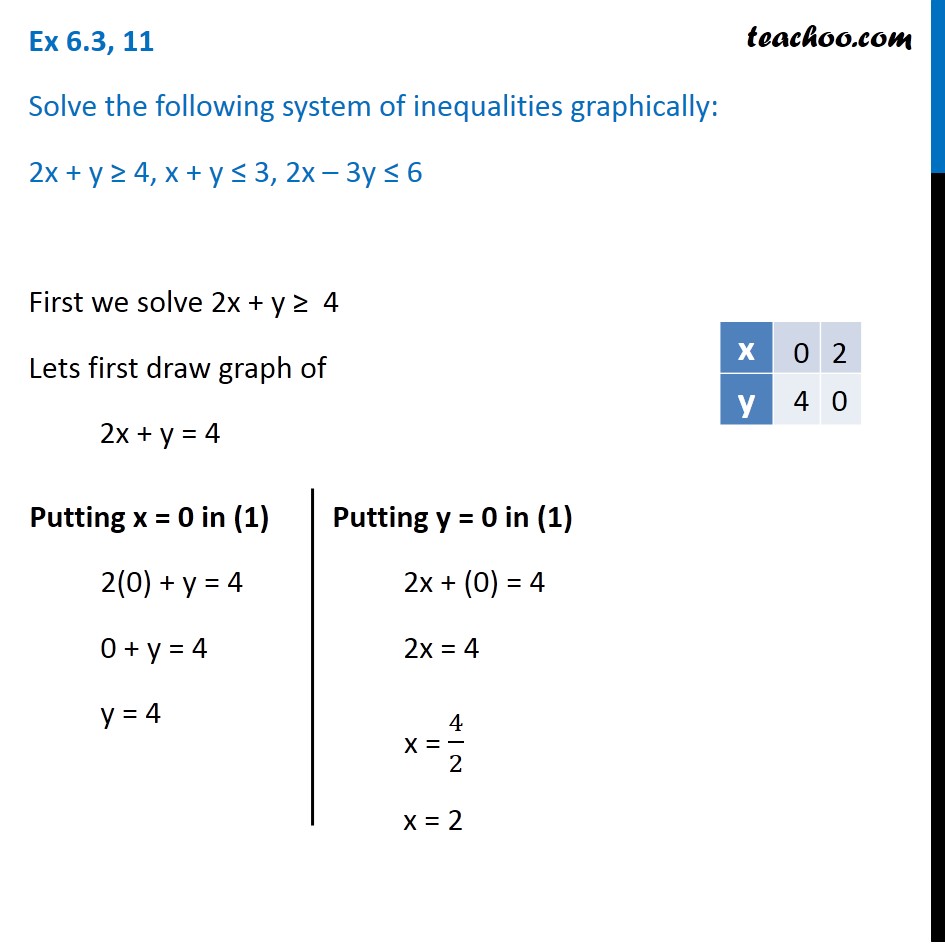
Ex 6 3 11 Solve 2x Y 4 X Y 3 2x 3y 6

Chapter 9 Systems Of Linear Equations Pdf Free Download

The Substitution Method

Math 432 Hw 2 5 Solutions Pdf Free Download

Graph Graph Equations With Step By Step Math Problem Solver

Systems Of Linear Equations

Solve These By Elimination Method 1 2x Y 4 And 5x 3y 1 2 Maths Pair Of Linear Equations In Two Variables Meritnation Com

Graphing Systems Of Linear Equations

The Equation Of Common Tangent To The Curves Y 2 4x And Xy 2

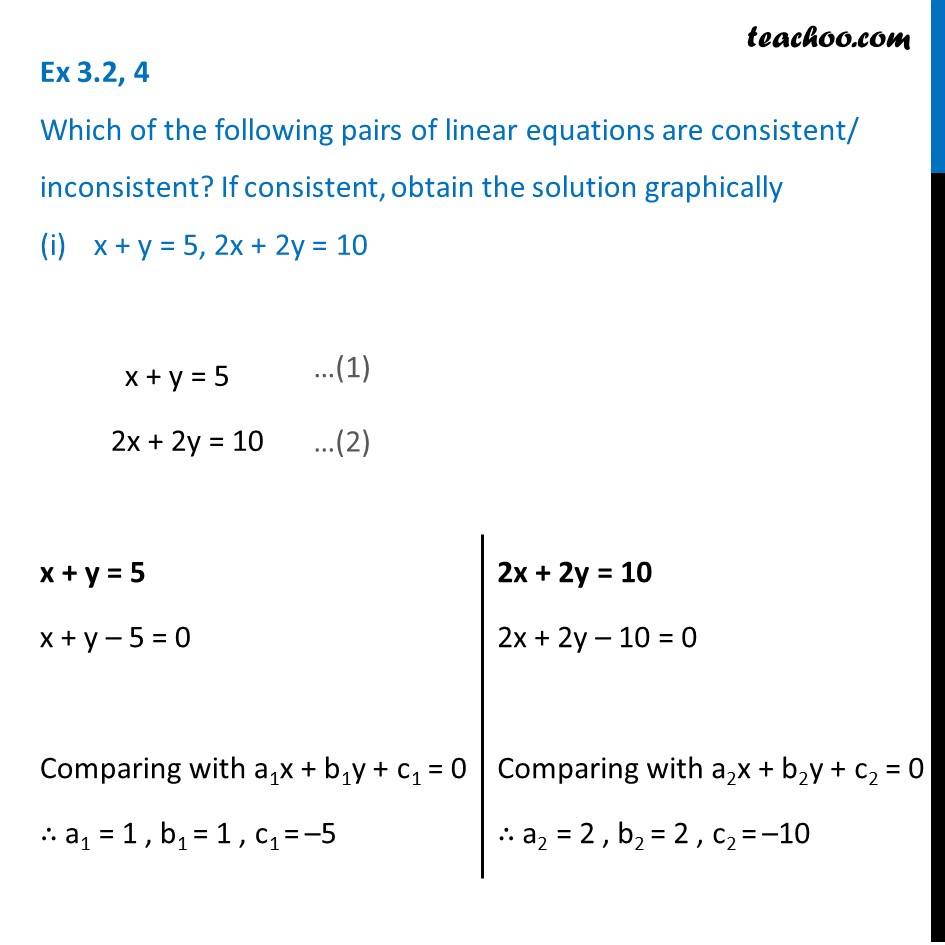
Ex 3 2 4 Which Of The Pairs Of Linear Equations Are Ex 3 2

Www Waynesville K12 Mo Us Cms Lib07 Mo Centricity Domain 603 A1 c6 l2 solution key worked out Pdf

Solve The Following Systems Of Equations 3 X Y 2 X Y 2 9 X Y 4 X Y 1 Sarthaks Econnect Largest Online Education Community

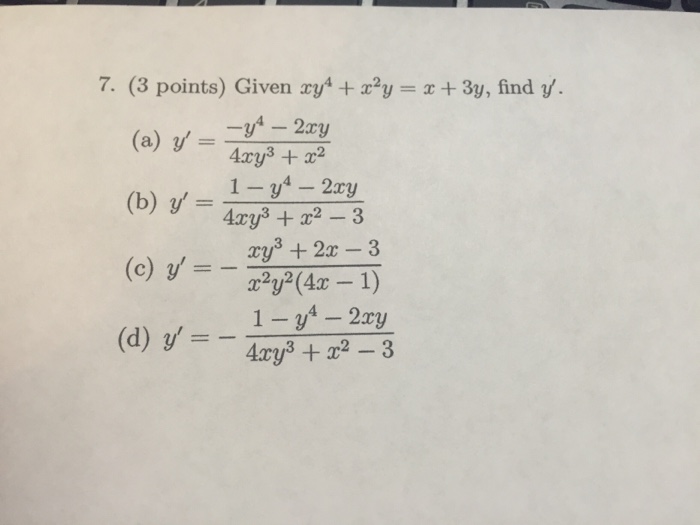
Given Xy 4 X 2y X 3y Find Y Y Y 4 2 Chegg Com

For Problems 26 Determine An Integrating Factor Chegg Com

What Is The Solution For Math Xy 2 2x 2y 3 Dx X 2y X 3y 2 Dy 0 Math Quora

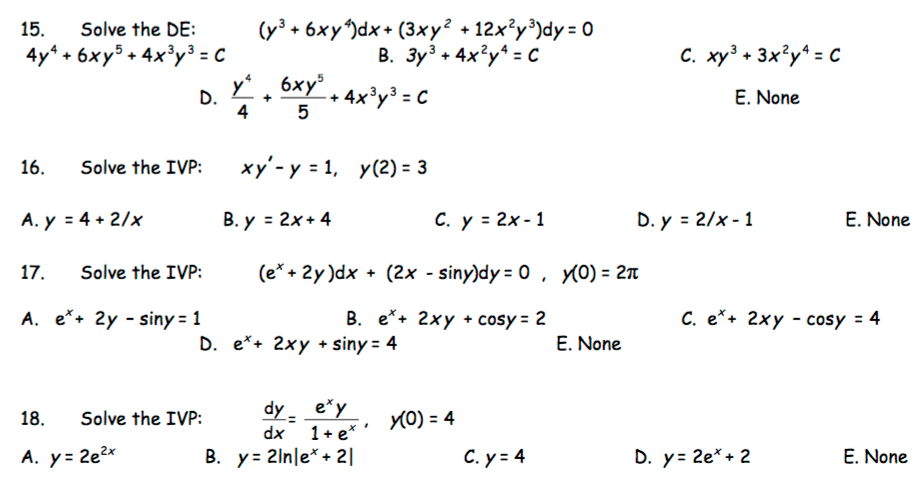
Solve The De Y 3 6xy 4 Dx 3 Xy 2 12x 2y 3 Dy Chegg Com

Graph Graph Equations With Step By Step Math Problem Solver

2 2x2y4 See How To Solve It At Qanda

1 3x2y 4 See How To Solve It At Qanda

Which Type Of Solution Will Equation X 2y 4 And 2x Y 5 Have Brainly In

3 Xy02x Y9 4 3x Y22x Y3 See How To Solve It At Qanda

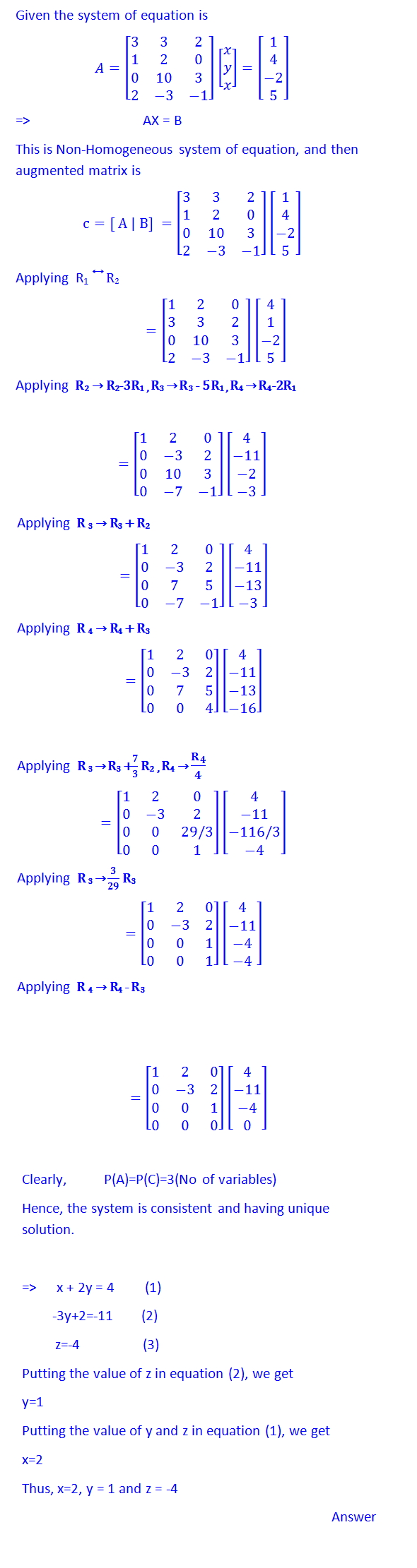
Solve The System Of Equations 3x 3y 2z 1 X 2y 4 10y 3z 2 And 2x 3y Z 5 Answer Mathematics 1 Question Answer Collection

16 1 2 Variables Separable Equations

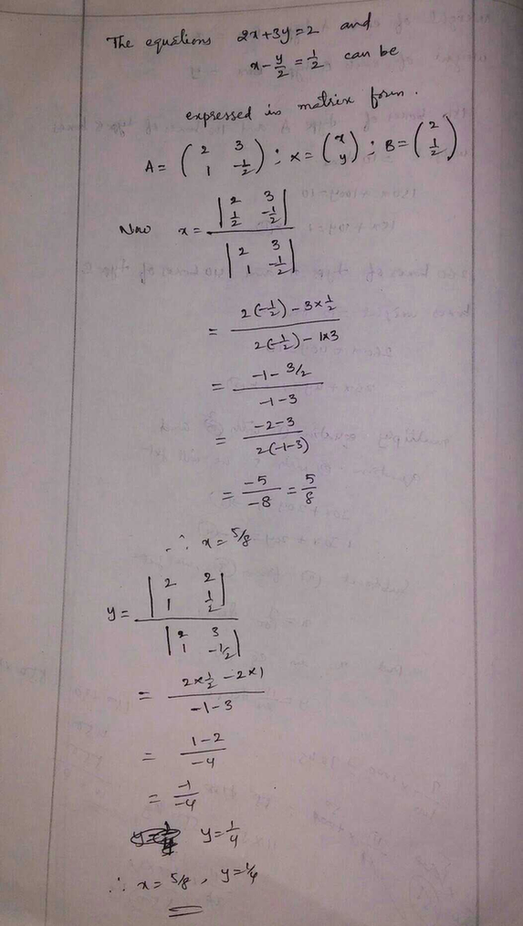
2x 3y 2 X Y 2 1 2 Using Cramer S Rule Scholr

X Y 14 X Y 4 Solve Using Substitution Method Youtube

Solutions To Implicit Differentiation Problems

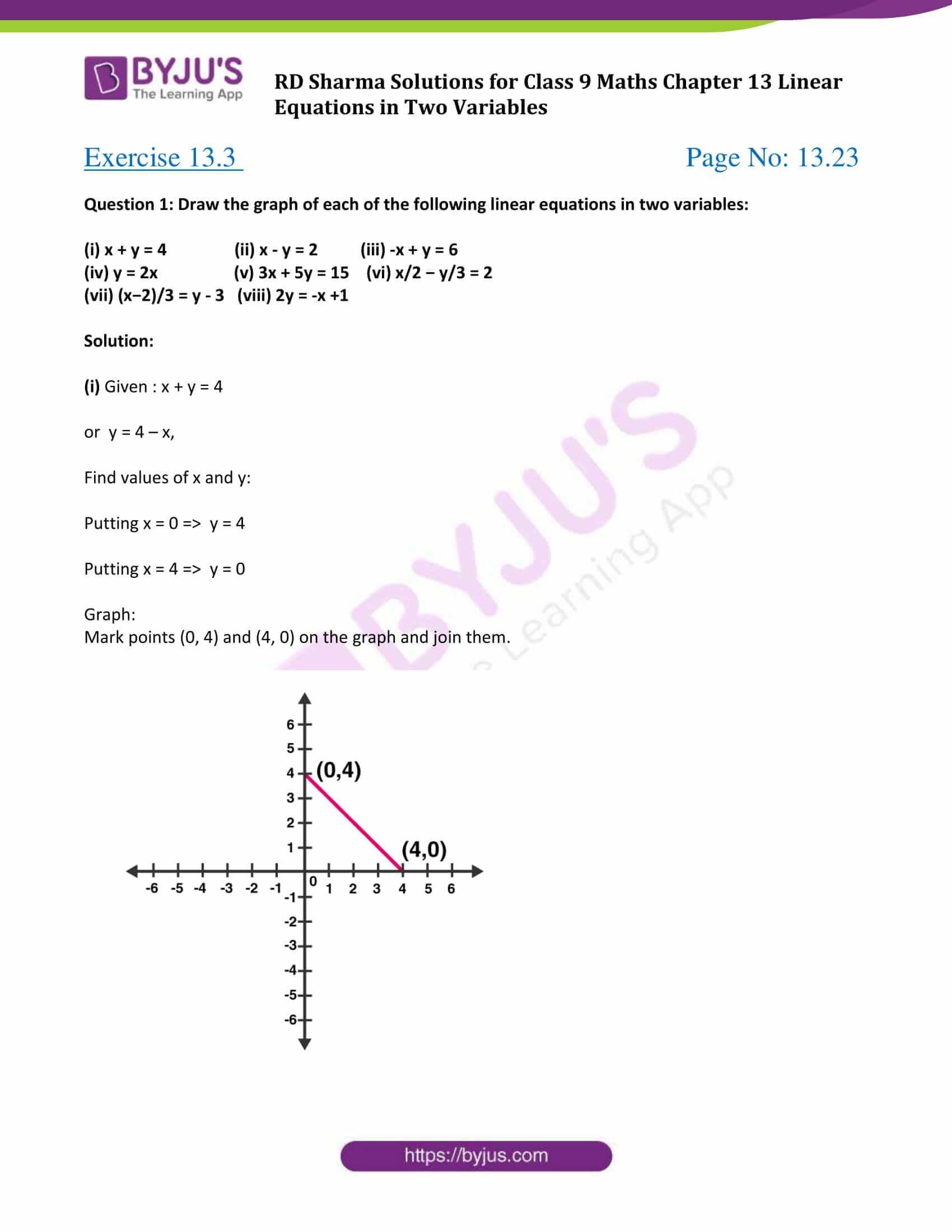
Rd Sharma Solutions Exercise 13 3 Chapter 13 Class 9 Linear Equations In Two Variables

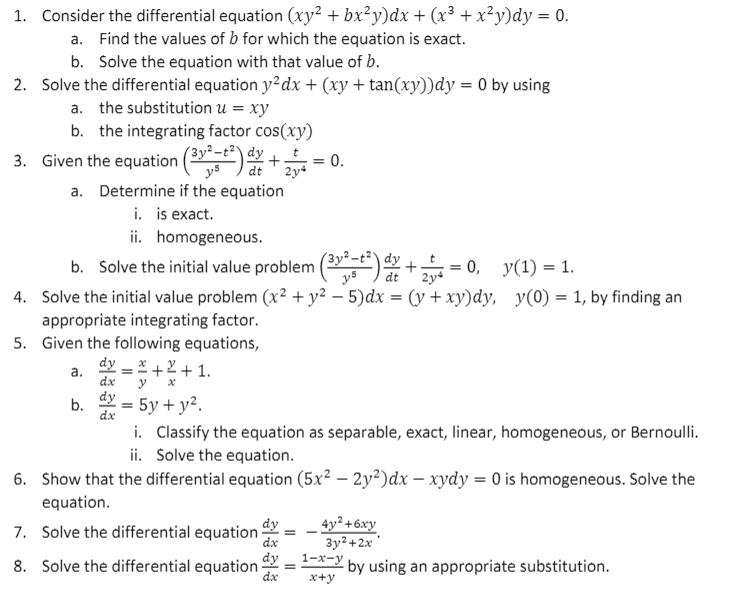
Consider The Differential Equation Xy 2 Bx 2y Dx Chegg Com

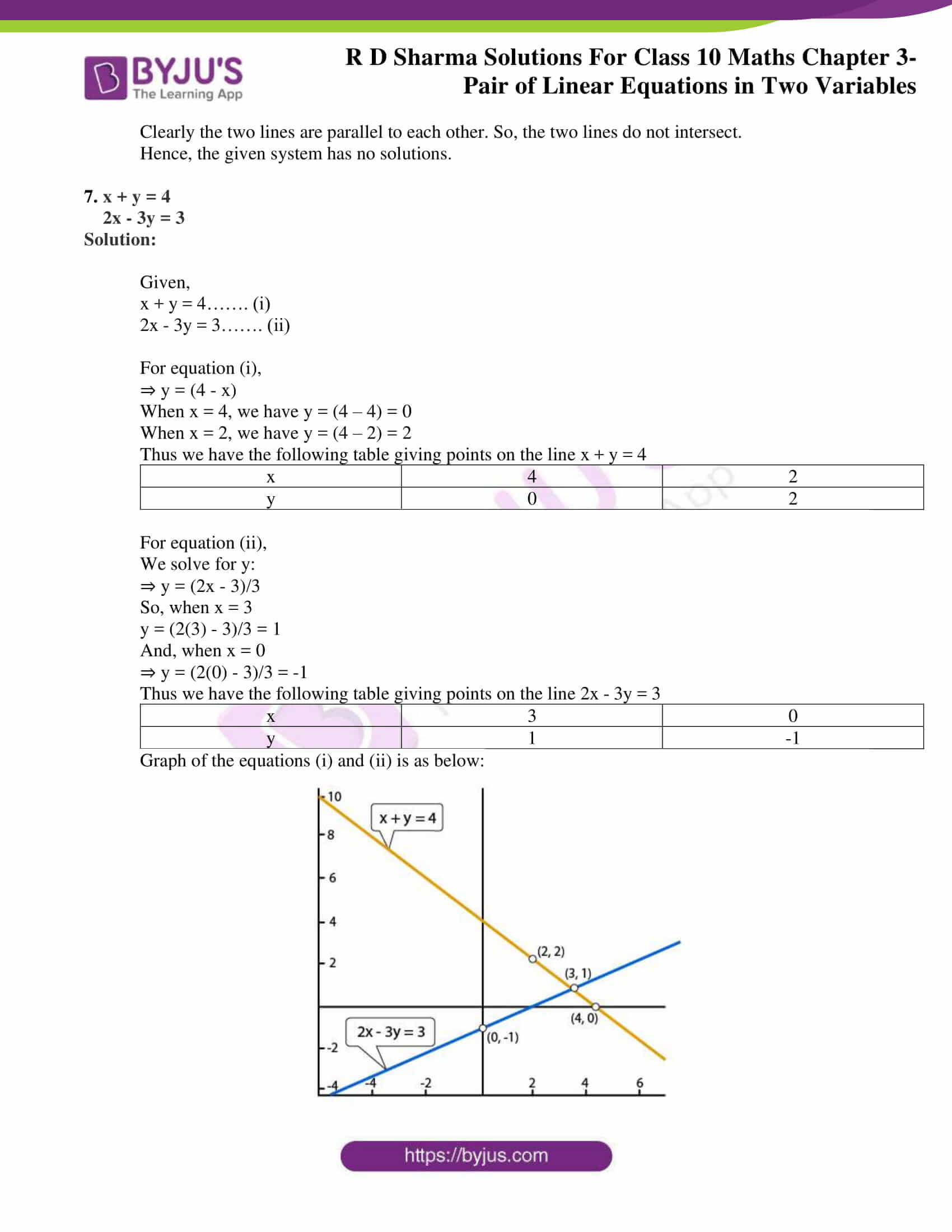
Rd Sharma Class 10 Solutions Maths Chapter 3 Pair Of Linear Equations In Two Variables Exercise 3 2

Lesson 6 Exact Equations Determine Whether Or Not Each Of

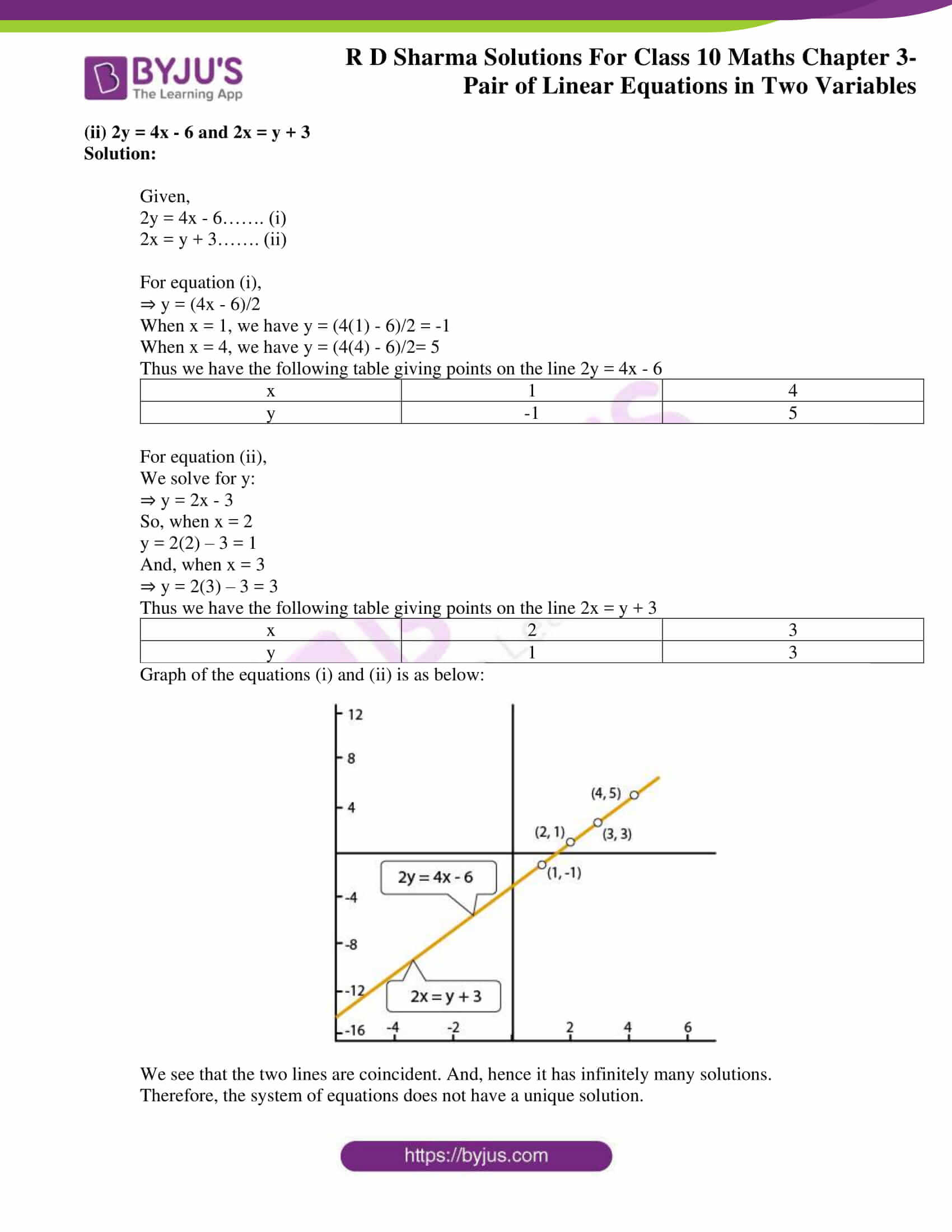
Rd Sharma Solutions For Class 10 Chapter 3 Pair Of Linear Equations In Two Variables

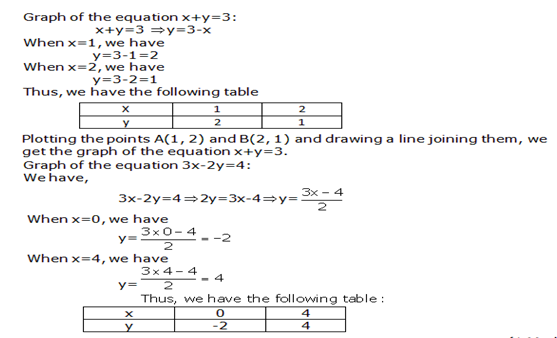
Solve Graphically The System Of Equations X Y 3 3x 2y 4 Mathematics Topperlearning Com Ja91bwemm